Horner’s Synthetic Division Method

We have learned to divide a polynomial (say, \(p(x)\)) by some other polynomial (say, \(g(x)\)) which is of degree lesser than or equal to the former one.

We use to call the method as long-division method of polynomial.

Let us recap it with an example.

Example: Divide \(3x^5-8x^4-5x^3+26x^2-33x+26\) by \(x^3-2x^2-4x+8\).
Solution:

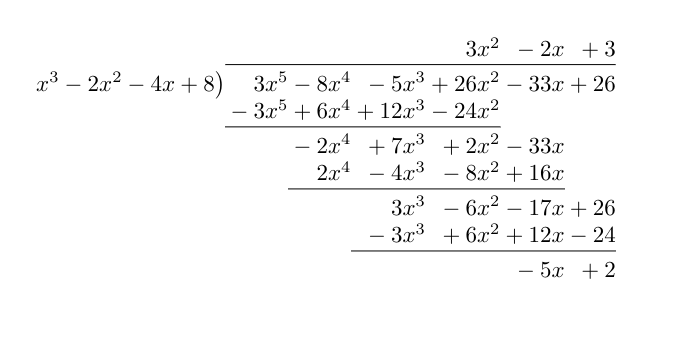
Attention

To be continued…
This article is under construction. It would be completed soon. Thank you.